Aero formulas
From NPrize
The List of elementary physics formulae on wikipedia is useful.
A page is dedicated to heat transfer.
List of variables
| Variable | Meaning | Unit (SI) |
|---|---|---|
| γ (gamma) | Surface tension or Heat capacity ratio (adiabatic process in thermodynamics) | N.m-1 (Newton per meter) |
| μ (mu) or η (eta) | Viscosity | Pa·s (Pascal second) or P (Poise, 1 Poise is 0.1 Pa.s) |
| ρ (rho) | Density | kg.m-3 (kg per cubic meter) |
| C, Cp, CV | Heat capacity, general, at constant pressure, at constant volume. | J.K-1 (Joule per Kelvin) |
| G | Gibbs free energy | J (Joule) |
| H | Enthalpy: total energy of a thermodynamic system. | J (Joule) |
| ΔHvap or L | Vaporization heat or Latent heat of vaporization: energy required to vaporize a mole of liquid at a given temperature. | J.mol-1 (Joule per mole) |
| M | Mach number | no unit |
| Q | Amount of Heat | J (Joule) |
| T | Temperature. T0 or Tt is the stagnation temperature. | K (Kelvin) |
| S | Entropy | J.K-1 (Joule per Kelvin) |
| U | Internal energy of a system (see first law of Thermodynamics below) | J (Joule) |
| V | Volume | m3 (cubic meter) |
| W | Work: mechanical constraints on the system. | J (Joule) |
| a | Speed of sound in medium (used to calculate Mach number) | m.s-1 |
| c | Velocity of a flow in thermodynamics, also noted V; generally noted u in fluid dynamics. | m.s-1 |
| n | Quantity of matter | mol (mole) |
| p | Pressure. pt is the stagnation pressure. | Pa (Pascal) |
| p | Momentum p = m*v, with m the mass and v the velocity, not to be confused with volume. | kg.m.s-1 |
List of constants
| Constant | Meaning | Value | Unit (SI) |
|---|---|---|---|
| NA or N | Avogadro constant, number of atoms or molecules in a mole. | 6.02214129.1023 | mol-1 |
| R | ideal gas constant | 8.3144621 | J.K−1.mol−1 |
| G | Gravitational constant | 6.674 | m3.kg-1.s-2 |
| kB or k | Boltzmann constant, gas constant R divided by Avogadro number. | 1.3806488.10-23 | J.K-1 |
List of equations
| Equation | Name | Meaning |
|---|---|---|

|
Ideal gas equation | Relation between properties of an ideal gas (state equation). k is kB. |

|
Clausius-Clapeyron relation | Relation between the pressure, latent heat of vaporization and temperature of a vapour at two temperatures (approximation, at low temperatures). |
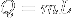
|
Heat at state change for an ideal gas. | The heat required to change the state of a some matter, L being the latent heat. Delta H equals Q only when pressure is constant (isobaric). |
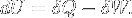
|
First law of thermodynamics | Variations of internal energy of a system between two states is the sum of the received heat and work (minus the given work). |
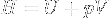
|
Enthalpy | Total amount of energy of a system, defined as the sum of the internal energy U of the system and pressure * volume at the boundary of the system and its environment. |
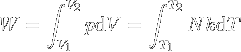
|
Work of gas expansion. | Work done by expanding an ideal gas. |

|
Internal energy change related to entropy | Internal energy related to entropy variation for a closed system in thermal equilibrium (fundamental thermodynamic relation). |
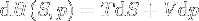
|
Enthalpy change | Enthalpy change depending on entropy and pressure changes, equation created from the mix of the basic ones above. |
| ΔSuniverse = ΔSsurroundings + ΔSsystem | Entropy variation as a whole. | Entropy variation of a system is generally compensated by the inverse variation of the surroundings, not including losses. |
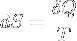
|
Second law of thermodynamics | A change in the entropy of a system is the infinitesimal transfer of heat to a closed system driving a reversible process, divided by the equilibrium temperature of the system. |
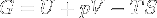
|
Gibbs free energy / Free enthalpy | Useful work obtainable from a system at isobaric and isothermal conditions. Since H is U + pV, it can be replaced in the equation, making G = H - TS. |
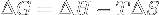
|
Gibbs free energy variation. | If ΔG < 0, the system's transformation can be spontaneous, if ΔG = 0 the transformation is inversible and the system is in an equilibrium state, if ΔG > 0 it can't be spontaneous. |
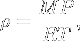
|
Density of an ideal gas. | M is molar mass. This means that the density of an ideal gas can be doubled by doubling the pressure, or by halving the absolute temperature. |